I probably should not have worn my frictionless shoes out last night.

Oddly enough (for the end of March) it’s snowing in State College, which means that the sidewalks are slippery again, which reminded me that I’ve been meaning to follow up my previous post about slippery surfaces with one where I talk about hills.

There were two main points I made in the last post:

1. friction with the ground is what allows you to accelerate (speed up, slow down, or change direction), and
2. friction also helps keep your feet from slipping out from under you when you put them down as you walk.

I used a slightly unrealistic diagram of a foot as a visual aid:

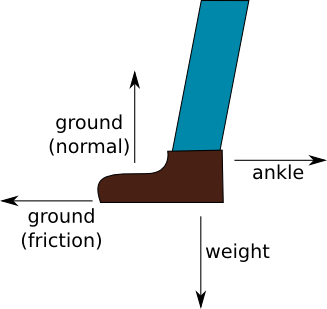
Now compare that to the equivalent diagram for a foot on a hill:

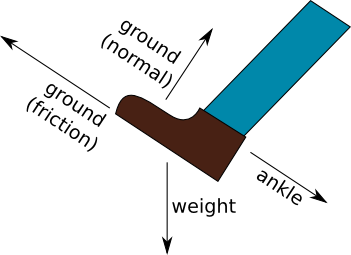
On level ground, without friction, there’s no force pushing you along the ground. But the new diagram shows that that isn’t the case anymore on a hill. With no friction, gravity works to pull you down the slope. So in the absence of friction, you do accelerate — you slip down the hill.

Obviously, this is bad news if you’re trying to walk uphill. Even if you’re not, though, you still have to worry about the new version of point #2: there’s a component of gravitational force actively “trying” to pull your feet out from under you every time you take a step. All this adds up to the fact that it’s just easy to lose control when walking on a slippery hill, period.

The one “loophole” in this argument is that just because there is a force pushing you down the hill, doesn’t mean you’re going to slide down the hill. Conservation of energy tells us that if you have some speed built up in advance, that will be enough to put you over a small enough hill. The equation is \(\frac{1}{2}mv^2 \ge mgh\), or \(v \ge \sqrt{2gh}\). Given that typical walking speeds are around \(\unit{1.4}{\frac{\meter}{\second}}\), you can get over a hill of… about \(\unit{10}{\centi\meter}\). Barely a bump.

If you’re thinking this is a silly calculation, well, you’re right. Of course you can walk over a hill higher than \(\unit{10}{\centi\meter}\). But then again, an icy surface is not really frictionless; typical values for the coefficient of friction between rubber and ice are in the range \(0.05-0.25\) depending on the exact details of the surface.

I’m going to use that to figure out something more interesting: what angle of inclination is safe to walk up? Newton’s second law applied to the hill-walking diagram above, assuming all of the person’s weight is on the foot shown, gives

(\(m\) is the person’s mass) in the direction normal to the slope, and

in the direction along the slope. Using the fact that \(f_s \le \mu_s F_N\), we get

This gives the maximum amount of force that your ankle can apply to your foot without it slipping — or equivalently, the maximum force that your foot can apply to your ankle to move your body forward. That force has to cancel out the parallel component of your weight to keep you moving uphill, so we need \(F_\text{ankle} \ge mg\sin\theta\), or after a bit of algebra,

For the high-end coefficient of friction, \(\mu_k \approx 0.25\) for rubber on dry ice, that gives about \(\unit{7}{\degree}\), and for slippery wet ice with \(\mu_k\approx 0.05\), you get \(\unit{1.4}{\degree}\). That’s a noticeable tilt, and a lot of roads are flatter than that, but slopes of more than \(\unit{7}{\degree}\) inclination and especially more than \(\unit{1.4}{\degree}\) are not that uncommon. So keep an eye out for them, and next winter (or, if you’re in the middle of Pennsylvania, tomorrow), you’ll know what roads to avoid.