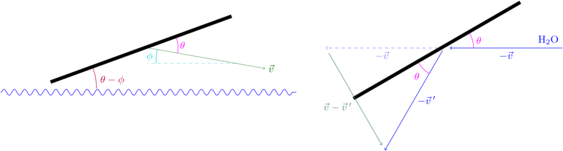
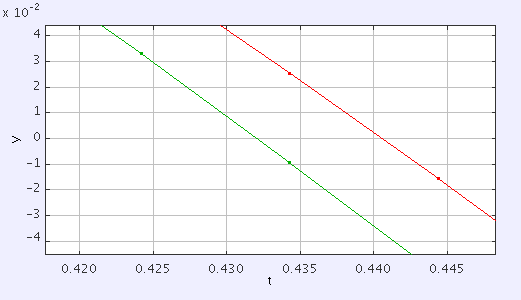
The Conifer Catapult
Posted by David Zaslavsky onThe tree catapult is a staple of cartoon physics, and I think I’ve even seen it acted out in one or two live-action movies. But according to the Mythbusters, it may have been actually used by medieval armies laying siege to castles. I actually thought preconstructed wheeled catapults would have been the norm, but still, you have to wonder: does it work?
The underlying concept behind a tree catapult is simple enough: trees are stiff. If you pull one down and let it spring back up, it stands to reason that you could potentially fling a dead body pretty far. But that very same stiffness that gives a tree its flinging power also makes it resist being pulled down in the first place. This is a pretty straightforward application of the principle of conservation of energy: whatever energy the tree is able to expend in flinging its cargo into the air has to come from the work you do while pulling it back in the first place.
I can make a rough calculation by treating the top of the tree as a spring, known in physics terms as a simple harmonic …